Fraction Tips and Tricks 1

Fractions are easy to learn if you know how to add and multiply WELL.

What Are Fractions?

A fraction describes to us a part of a whole number (1, 2, 3, 4…) or a group of numbers. Fractions are the numbers between 0 and 1, or 1 and 2, etc.. They result from a division of a whole number.

Let’s take a look at the two numbers that make up a fraction.

Sometimes it’s hard to remember numerators from denominators. Try letting the “D” in denominator remind you of the “D” in down. Likewise, the “U” in numerator can remind you of the “U” in up.

Use the space below to label the parts of this fraction.

The upper number is the _______________ and the lower number is the _________________. We would read this fraction as ______-________.

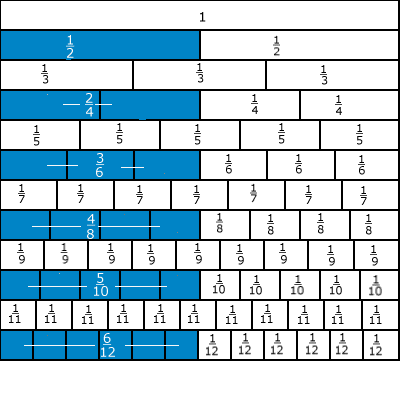
Below is a picture of how different fractions can divide the number 1.

Again, the numerator is the number being divided and the denominator tells us how many times to divide it. In the example above, the numerator one (1) was divided by denominators 2 – 12 .

This means we took one thing and divided it 2, 3, 4, 5, 6, 7, 8, 9, 10, 11 and 12 times. The picture above also illustrates that the size of the boxes equal the quotient size.

We also should notice an important thing about fractions which is: with a fixed numerator, the bigger the difference between the numerator and denominator the smaller the fraction.

For example, look at the above picture of different fractions….you’ll see that the 1/2 boxes and 1/12 boxes are much different in size. This shows that the fraction 1/12 is much smaller than 1/2. 1/12 has a bigger difference in sizes between the numerator and denominator.

Use the spaces below to make your own fractions of 1.

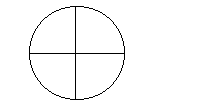
Here we divided a circle into 4 parts. We then shaded areas of it to give us the fractions 1/4, 2/4, 3/4.

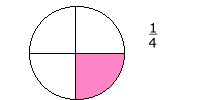
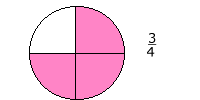
Shade and label the fractions in the picture below.

So, let’s summarize what we know about fractions so far.

• Fractions describe part of a number or group of numbers.
• Fractions have two parts, the numerator and the denominator
• The numerator is the top number, the denominator is the bottom number
• The denominator divides the numerator.
• Fractions are divisions.

Here is a fractions worksheet to help you identify fractions.

As you can see from the problems above, we are moving to a higher level in math. To continue learning fractions, we need to detour a bit and learn how to factor whole numbers and understand prime numbers, greatest common factors and least common multiples. This will aid us in comparing, reducing, adding, subtracting, multiplying and dividing fractions. The Tips section factor whole numbers shows important skills for future math operations. Then, come back to this point again and continue to learn about fractions.

Equivalent Fractions

Let’s take a look at equivalent fractions. Equivalent fractions say the same thing in different ways. For example,

As you know 2 is half of 4 and 1 is half of 2….50 is half of 100, all of these fractions = 1/2 (one-half) because they all say or describe the same thing in different ways. We call these equivalent fractions.

Here are more examples of equivalent fractions.

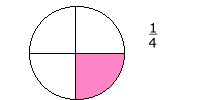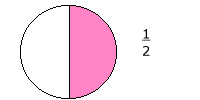Here is another look at the picture above with some of the equivalent fractions highlighted.

Can you find other equivalent fractions in the picture above?

For example:

• Are 1/4 and 2/8 equivalent?
• Are 2/5 and 4/10 equivalent?
• Are 1/3 and 3/9 equivalent?

Let’s take a look at these pictures.

The picture on the left shows 1/4. The picture on the right shows 2/8. As you can see, both of the fractions have the same amount of area shaded. This indicates that the fractions are equivalent.

Finding Equivalent Fractions

To find out if two or more fractions are equivalent, we use an operation called cross-multiplication. To cross-multiply, take the first numerator and multiply it by the second denominator. In the problem below, we’d multiply 1 and the 12 first and write the product (12) as a numerator.

Next, multiply the first denominator 3 and the second numerator 4 and write that product 12 as a denominator as shown below. As a result, we end up with the product 12/12.

Since the numerator and denominator of the product 12/12 are equal/identical we have found two equivalent fractions 1/3 and 4/12. This can be seen by comparing shaded areas in the example below.

Equivalent fractions happen when the fractions are multiples of the same number.

For example:

In summary, if you want to know if two or more fractions are equivalent, cross-multiply the numerators and denominator and if the product results with a fraction of identical numerators and denominators, the fractions are equivalent.

But, if the product of a cross-multiplication results in a fraction without identical numerators and denominators the fractions are NOT equivalent as can be seen in the next example below.

Let’s check to see if the fractions 1/2 and 1/6 are equivalent.

Use the cross-multiplication discussed above to cross-multiply the fractions shown below.

We begin by multiplying 1 × 6 for a product of 6 and write that as either the numerator or denominator.

Next, we multiply 1 × 2 for a product of 2 and write that in as shown below.

As you can see, the product of the cross-multiplication 1/2 and 1/6 is 6/2. 6/2 does not have identical numbers as both numerator and denominator. So the above fractions (1/2 and 1/6) are not equivalent.

Now here’s a chance for you to find equivalent fractions and to try cross-multiplication.

Use cross-multiplication on the problems below to find equivalent fractions.

Insert another equivalent fractions exercise here.

Comparing Fractions

So far we’ve learned about what fractions are and how to find equivalent and non-equivalent fractions. We noticed that fractions can have a wide variety of numerators and denominators depending on a fraction’s size. It’s easy to tell from some of the examples above that 1/2 is larger than 1/3. But, it’s not so easy to tell if the fraction 14/23 or if 12/25 is larger.

So when we want to know how big or small one fraction is compared to another we need to compare fractions. We know from above that if we cross-multiply two fractions and find a product with identical numerators and denominators that the fractions are equivalent. Likewise, if the product of cross-multiplying fractions results in a product with unlike numerators and denominators that the fractions are not equivalent. To compare fractions, we use the same cross-multiplication steps shown above to see which fractions are larger or smaller.

This is how to compare fractions:

• If the denominators are the same, the fraction with the larger numerator is the larger fraction. For example, 2/4 and 3/4 both have the same denominator, 4. So we look at the numerators and see that one is 2 and one is 3. If we following the rule above, we see that 3/4 is larger than 2/4 just because 3/4 has a larger numerator than 2/4.
• If the denominators are different. then use the cross-multiplication steps listed in the equivalent fractions section above.

Let’s begin comparing 14/23 and 12/25 by multiplying the first numerator (14) with the second fraction’s denominator (25). Write the result (350) as a numerator as shown in the example below.

Next complete the cross-multiplication by multiplying the first denominator (23) with the second fraction’s numerator(12) and write the result (276) as the denominator as shown below.

Now, really pay attention!!!….the fraction 350/276 shows that the first fraction 14/23 is larger than 12/25. How this works is by sequencing of multiplication and the size of the cross-products.

Let’s review the steps again below.

Let’s do another example comparing the fractions 5/13 and 6/14.

First, cross-multiply 5 and 14 write the result 70 as a numerator like below.

Now cross-multiply the 13 and the 6 and write the result 78 as a denominator as shown below.

This example shows that the second fraction 6/14 is larger than the first fraction 5/13.

In summary, the numerator of the third fraction shows the size of the first fraction and the denominator of the third fraction shows the size of the second fraction….I hope this isn’t too confusing.!???!!!????

If you cross-multiply in the sequence shown above, and the numerator of the third fractions is largest, the first fraction is largest. If the denominator of the third fraction is largest then the second fraction is largest…..get it??????

To check if these are accurate, divide each of the fractions using a calculator and the one with the largest quotient is the larger fraction.

Using the fractions 5/13 and 6/14 first divide 5 by 13 and the quotient is .385. Now divide 6 by 14 and the quotient is .429. And we know that .4 is larger than .3.

Converting Fractions

As we move along in fractions we have to gain more tools for dealing with fractions and one of those tools is called converting fractions. What this means is that we can take a fraction like 1/2 and turn it into 2/4, 3/6 or 4/8 by multiplying different non-zero numbers to both the numerator and denominator as shown below.

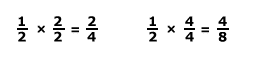
As you can see from above, we multiplied 1/2 to 2/2 and came up with 2/4. All we do is multiplied the numerators 1 × 2 straight across for a converted fraction numerator of 2. Then we take the denominators 2 × 2 and multiplied them straight across as the converted fraction denominator 4. (Psssst, now you know how to multiply fractions, too.)

Now if we cross-multiply to see if 2/4 is equivalent to 1/2 we’ll see that it is.
In the other example above, we took the fraction 1/2 and multiplied 4/4 to it and came up with the product 4/8. The only thing you have to remember is make sure you use the same number for the numerator and denominator and multiply straight across to convert any fraction to another equivalent fraction.

Hey Rick, insert an activity here!

Reducing Fractions

Reducing fractions is just the opposite of converting fractions. What we do is factor out the multiples common to both the numerator and denominator if we can. We factor out (divide out) the greatest common factor of both the numerator and denominator. This will result in a equivalent fraction of lowest terms or what we call a reduced fraction.

So here is how to reduce fractions.
As shown below, the fraction 2/4 is being divided by the greatest common factor of 2 and 4 which is 2. The resulting quotient is the fraction 1/2 which is in the lowest terms since its numerator and denominator is divisible by itself and 1.

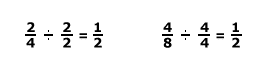
As seen above, all we do is divide straight across. Begin by finding all the factors of 2 and 4. If we remember our greatest common factor lesson we know that all the factors of 2 are 1, 2 and the factors of 4 are 1, 2 and 4. The greatest common factor shared by both 2 and 4 is 2. So we divided 2/4 by 2/2 and reduced it to 1/2. We did the same thing to 4/8. We find the greatest common factor of 4 and 8 which is 4 and we divided 4 out of the fraction and reduced it to 1/2.

Below are several problems you can practice on.

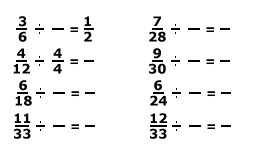
Remember, the most important thing is to find a factor that will divide BOTH THE NUMERATOR AND DENOMINATOR if possible. The reason we say “if possible” is because we could run into fractions with prime numbers in the numerator or denominator that can’t be factored any further such as those shown below.

Improper Fractions

So far, we’ve been dealing with fractions that have numerators of lesser value than denominators. Or top numbers that are smaller than the bottom numbers.
For example:

See how the numerators are smaller than the denominators?

Improper Fractions have larger numerators than denominators.

Below are some examples of improper fractions:

In the area below, identify and circle the improper fractions:

Rick, insert improper fractions exercise here!

Above all, remember that improper fractions are useful when we add, subtract, multiply and divide fractions as you’ll see further down this page.

Mixed Numbers

Mixed numbers are fractions that have both a whole number and a fraction. Below are some examples of mixed numbers.

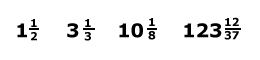
As you can see there are both whole numbers (in this case, 1, 3, 10 and 123) and fractions (1/2, 1/3, 1/8 and 12/37). Mixed numbers are very common in fractions and we’ll be seeing more of them as we add, subtract, multiply and divide fractions and work with improper fractions.

Let’s see if you can identify the mixed numbers in the area below.

Identify and circle the mixed numbers and draw a box around the improper fractions.

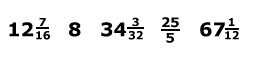Rick, insert mixed number exercise here.

Converting Mixed Numbers
To Improper Fractions

In this section we’re going to learn how to convert mixed numbers to improper fractions. This is a very important skill to know when adding, subtracting, multiplying and dividing fractions.

How to Convert Mixed Numbers to Improper Fractions

Converting a mixed number to an improper fraction is easy.

First, multiply the denominator of the fraction to the whole number.

Then add the numerator to the product of the multiplication and move the denominator to the new numerator. The result will be an improper fraction.

Here’s an example of converting the mixed number 41/2 to the improper fraction 9/2:

Converting Improper Fractions To Mixed Numbers

This is very easy to do! Just divide the numerator by the denominator and you get a whole number and a regular fraction. In the example below, we’ll take the improper fraction 7/3 and convert it to the mixed fraction 21/3.

Go to Fractions Tips and Tricks Page #2