Areas: Polygons and Circles

First Glance

What is area?

You may come across the term “area”. Have you ever wondered what area is all about? It is the available space within a fixed boundary. In mathematical terms, area is the calculation of the number of square units required to cover up a shape area. Moreover, the unit of area is always associated with a square as area is always a multiplication of two units.

There are different ways to calculate an area. You can take the help of drawing a shape on the graph area and then count the number of squares inside the shape and calculate the area but it is not always an effective method. Therefore, it is always better to use formulas, which presents a scientific process to calculate the area. The polygons and the circle, both have its own formulas to calculate the exact area.

During the calculations of many geometric shapes you may come across terms like  (pi). You need not worry at all as the value of  remains same trough out that is 22/7. Some of the common formulas to calculate the area of various geometric figures are as follows:

Square

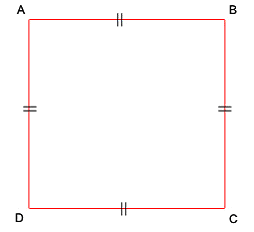
Area of a square = s², where s = side.

 

Rectangle

Area of a Rectangle = l x h, where l = length and h = height

 

Triangle

Area of a triangle = ½ (b x h), where b = base and h = height

Parallelogram

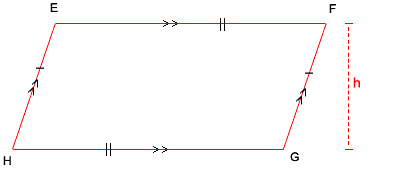
Area of a parallelogram = l x h, where l = length and h = height.

Circle

Area of a circle: A=  r2, where  = 22/7, r = radius.

Details

Calculating area is very much necessary in every aspect of our life. For instance, if you want to know the floor area of an apartment or the area of a plot, you will have to know it in terms of surface area. Apart from this, many other aspects need to be revealed in terms of area. Area is actually the number of square units that is needed to cover a shape. Therefore, you need to be aware of the particular formulas that are required to calculate the areas of these shapes.

Area of a Rectangle

One of the simplest polygons is the rectangle with the opposite sides equal to each other. It has two dimensions, length and height.  To find out the area of a rectangle all you need to do is, length x height. Suppose the length of the above rectangle is 5 feet and the height is 3 feet, then the area of the rectangle will be 5 feet x 3 feet = 15 square feet. The height is also referred as “width” or “altitude”.

Area of a Square

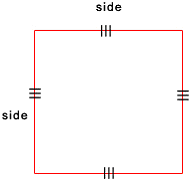
A square is also a simple polygon and you can easily find the area of a square. The square possesses 4 sides of equal sizes so there is no distinction between length and height. Therefore, the area of a square = side x side = side². Suppose the above square has sides which measures around 5 feet then the area of the square will be = 5². The area of the square will be = 25 square feet.

Area of a Parallelogram

Whenever, you observe a parallelogram, you will find a similarity with rectangle. The only difference is that the interior angles of a rectangle are all right angles but in case of parallelograms, they are not. However, the change in angle has not brought any change to the formula of calculating the area of parallelogram. The formula is the same as that of the rectangle, length x height. Therefore, if a parallelogram has 8 cm of height and 14 cm of length then the area of the parallelogram will be 8cm x 14cm = 112 cm².

Area of a Triangle

A triangle is another polygon, which has three sides. There is a particular formula for measuring the area of a triangle as well. You can measure the area of a triangle by using the formula ½ (b x h). If you consider a triangle ABC, and draw a perpendicular AD to the base BC then you can consider AD as the height. Suppose if the triangle has a base BC, measuring 6cm and the height AD, 8cm then the area of the triangle is ½ (base x height).

Area of Triangle ABC = ½ (6 x 8)
Area = ½ (48)
Area = 24 cm²

Area of a Trapezoid

You may get little bit confused in calculating the area of a trapezoid. However, once you know the correct way it will be a very easy process for you. All you need to do is draw a diagonal and divide the trapezoid into two halves. Once you divide the trapezoid, you will get two bases along with the two triangles. Name the two bases as base 1 and base 2. Suppose, there is a trapezoid EDGF is a trapezoid. If you draw a diagonal from G to E then the diagonal GE divides the trapezoid into two triangles, triangle FEG and triangle EDG having base 1 and base 2 respectively. The height is same in both the cases. Therefore, once the base and height of the triangle is known, you can easily calculate the area of the trapezoid by adding the area of the two triangles.

Suppose, base 1 of the triangle FEG is 6 cm and base 2 of the triangle EDG is 10 cm. and the height is 4 cm in both the cases. Then the way to find out the area of the trapezoid is,

Area = area of triangle FEG + area of triangle EDG
Area =  ½ (4 x 6) + ½ (4 x 10)
Area = ½ x 24 + ½  x 40
Area = 12 + 20
Area = 32 cm²

Area of a Circle

Area of a circle is a bit different from calculating the area of a polygon. It involves a simple formula, which needs only the measurement of the radius of the circle. The formula is r² is a constant value so you need not worry at all to calculate the area of a circle. For instance, if the above circle has a radius of 7 cm then the area of the circle will be

Area = 22/7 x 7²
Area = 22/7 x 7 x 7
Area = 22/7 x 49
Area = 22 x 7
Area = 154 cm²

Examples

Referring to the above rectangle ABCD, where its side BC = 6 cms and DC = 8 cms, its area would be:
Area of a rectangle = length x breadth
Area of a rectangle ABCD = 8 x 6
Area of a rectangle ABCD = 48 cm²

Referring to the above square ABCD, where its side DC = 4 cms, its area would be:
Area of a square = side²
Area of a square ABCD = 4²
Area of a square ABCD = 16 cm²

Referring to the above Trapezoid ABCD, where one of its side DC = 14 cms, side AB = 6 cms and height is 12 cms, its area would be:
Area of a trapezoid = ½ (b x h) + ½ (b x h)
Area of a trapezoid ABCD = ½ (14 x 12) + ½ (6 x12)
Area of a trapezoid ABCD = 84 + 36
Area of a trapezoid ABCD = 84 + 36
Area of a trapezoid ABCD = 120 cm²

Referring to the above Circle, where its radius = 6 cms, its area would be:
Area of circle =  r²
Area of circle = 22/7 (6²)
Area of circle =22/7 x 36
Area of circle = 18.9 cm²

Test your knowledge – try our Polygons and Circles Area Test.